Flight through Schoen’s Gyroid Minimal Surface

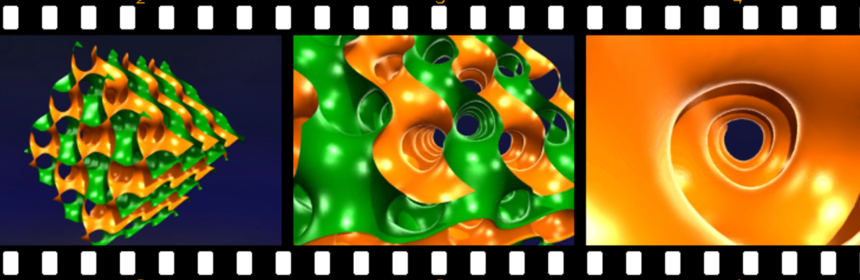
The cubic Gyroid surface is a “bicontinuous” minimal surface that divides space into two intertwined network- or labyrinth-like subdomains. The two subdomains are enantiomorphic, that is identical apart from different handedness. This animation shows a rotation of a fairly large subsection of this periodic surface, and a flight through one of the two domains. The flight path first follows a three-fold screw axis, and then turns into a four-fold screw axis. In a second run of the same animation, the same surface is shown together with the skeletal graphs that are often used to represent the Gyroid.

Movie files: AVI

If you are using this animation, please reference the publication that describes it: Hyde & Schröder-Turk, Geometry of interfaces: topological complexity in biology and materials, Interface Focus 2(5), 529-538 (2012)

This animation was produced at the ANU during my PhD. Special thanks to Stuart Ramsden for a lot of help!

A few different formats are available from these dropbox links:

https://www.dropbox.com/s/ko1xqzk6k63463o/GyroidFlyThruSchroederTurk.avi?dl=0

https://www.dropbox.com/s/o4nu0dqu66426ua/GyroidFlyThruSchroederTurk_reasonable.mp4?dl=0

https://www.dropbox.com/s/ceudal5pba8ngyg/GyroidFlyThruSchroederTurk_verySmall.mp4?dl=0