Front Page News! Copolymeric meltdown due to geometric frustration

Well done, Tobias Hain, for the publication of your first research paper. A lovely piece of simulation work, carried out together with Jacob Kirkensgaard, on geometric mechanisms at play when copolymers self-organise on a spherical substrate. Not only did the pretty pictures tickle the interest of Softmatter’s front page department, but the message resonated with a member of the public… who noted

“I actually had a copolymeric meltdown in front of my kids this morning due to geometric frustration, so looking very much forward to study the paper” (Johan R.)

Further, the publication also came to the attention of the Journal of Nutrition and Obesity whose editors seemingly found the study “exceptionally interesting”.

These interdisciplinary aspects aside, Tobias’ paper addresses what happens when star copolymers, with three mutually immiscible arms, assemble on a spherical substrate. The copolymer’s desire to form hexagonal honeycombs (as the optimal structure on a planar substrate) has to compete w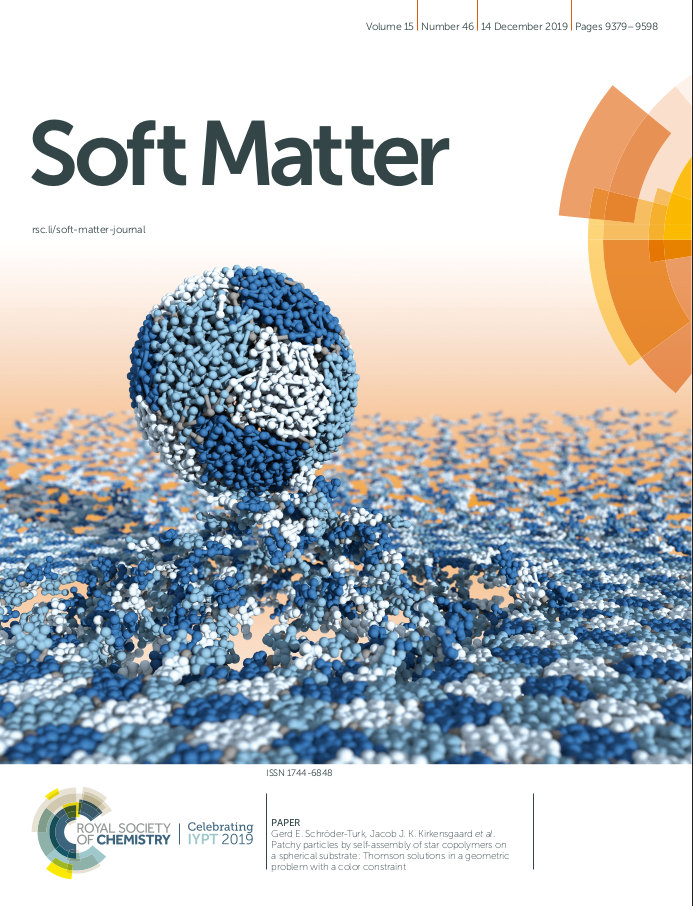ith color constraints and topological defects that emerge on a sphere. The research, led by Jacob Kirkensgaard, was published in Soft Matter:

T. Hain, G.E. Schröder-Turk and J.J.K. Kirkensgaard, “Patchy particles by self-assembly of star copolymers on a spherical substrate: Thomson solutions in a geometric problem with a color constraint“ (arxiv version here), Soft Matter, 2019, 15, 9394-9404

The abstract is here: Confinement or geometric frustration is known to alter the structure of soft matter, including copolymeric melts, and can consequently be used to tune structure and properties. Here we investigate the self-assembly of ABC and ABB 3-miktoarm star copolymers confined to a spherical shell using coarse-grained dissipative particle dynamics simulations. In bulk and flat geometries the ABC stars form hexagonal tilings, but this is topologically prohibited in a spherical geometry which normally is alleviated by forming pentagonal tiles. However, the molecular architecture of the ABC stars implies an additional ‘color constraint’ which only allows even tilings (where all polygons have an even number of edges) and we study the effect of these simultaneous constraints. We find that both ABC and ABB systems form spherical tiling patterns, the type of which depends on the radius of the spherical substrate. For small spherical substrates, all solutions correspond to patterns solving the Thomson problem of placing mobile repulsive electric charges on a sphere. In ABC systems we find three coexisting, possibly different tilings, one in each color, each of them solving the Thomson problem simultaneously. For all except the smallest substrates, we find competing solutions with seemingly degenerate free energies that occur with different probabilities. Statistically, an observer who is blind to the differences between B and C can tell from the structure of the A domains if the system is an ABC or an ABB star copolymer system.

Much of this work was conducted while both Tobias and I had a fabulous time at Copenhagen and Lund University, in the first half of 2019. I am very grateful to the Danish National Bank “Nyhavn 18 scheme”, the Camurus Lipid Research Foundation and the Natural Science Faculty at Copenhagen University who made my stay possible, and to the Physical Chemistry Department of Lund University who supported and hosted Tobias. Very grateful.